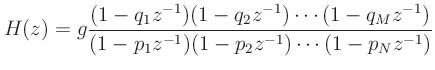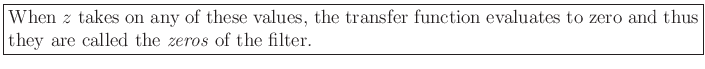Poles and Zeros cont.

The factored transfer function is given by

$\displaystyle H(z) = g\frac{(1-q_1z^{-1})(1-q_2z^{-1})\cdots(1-q_Mz^{-1})} {(1-p_1z^{-1})(1-p_2z^{-1})\cdots(1-p_Nz^{-1})}$

Zeros

The roots of the numerator polynomial are given by

$\displaystyle \{q_1, q_2, \ldots, q_M \}.$

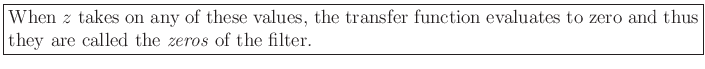
$\fbox{\parbox{6in}{When $z$\ takes on any of these values, the transfer function evaluates to zero and thus they are called the \emph{zeros} of the filter.}}$

Poles

The roots of the denominator polynomial are given by

$\displaystyle \{p_1, p_2, \ldots, p_M \}$

$\fbox{\parbox{6in}{When $z$\ approaches any of these values, the transfer functi... ...r, approaching infinity. Thus these are called the \emph{poles} of the filter.}}$

``Mus 270a: Introduction to Digital Filters'' by Tamara Smyth, Department of Music, University of California, San Diego.

Download PDF version (filters.pdf)
Download compressed PostScript version (filters.ps.gz)
Download PDF `4 up' version (filters_4up.pdf)
Download compressed PostScript `4 up' version (filters_4up.ps.gz)