Why Spectral Notches?

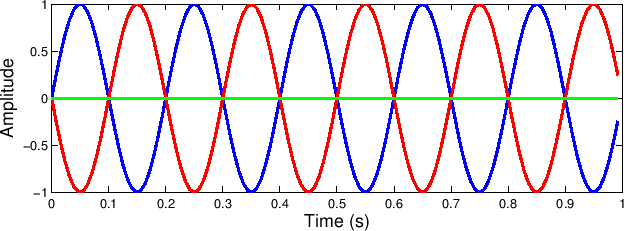
Notches occur in the spectrum as a result of destructive interference.

Recall that delaying a sine tone 180 degrees (1/2 a cycle) and summing with the original will cause the signal to disappear at the output.

Figure 17: Complete destructive interference.

If a sinusoid has a frequency of 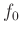$f_0$, then a delay of 1/2 cycle corresponds to a delay of

$$\tau = \frac{1}{2f_0}$$    seconds

or

$$M = \frac{f_s}{2f_0}$$    samples$$.$$