Distortion Index

If the input cosine has an amplitude of $a$, then the output in polynomial form becomes

$$F(ax) = d_0 + d_1ax + d_2a^2x^2 + ... + d_Na^Nx^N$$

Example: Given the waveshapping transfer function

$$F(x) = x + x^3 + x^5,$$

an input sinusoid with amplitude $a$ yields the output

$$F(ax) = ax + (ax)^2 + (ax)^5,$$

with the amplitude of each harmonic calculated using Pascal's triangle to obtain

$$h_1(a) = a + \frac{1}{4}3a^3 + \frac{1}{16}10a^5$$

$$h_3(a) = \frac{1}{4}a^3 + \frac{1}{16}5a^5$$

$$h_5(a) = \frac{1}{16}a^5$$

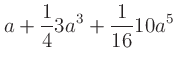
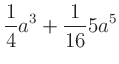
Because an increase in $a$ (typically between 0 and 1) produces a richer output spectrum, it is often referred to as a distortion index (analogous to the index of modulation in FM synthsis).