If 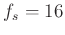 , what is the frequency of the sinusoid?
, what is the frequency of the sinusoid?
Method 2: the period of the sinusoid is
and the frequency is
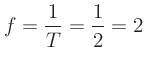
Hz
If 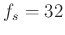 , then the frequency is higher:
, then the frequency is higher:
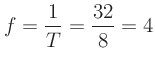
Hz
``Music 171: Fundamentals of Digital Audio''
by Tamara Smyth,
Department of Music, University of California, San Diego (UCSD).
Download PDF version (digitalAudio171.pdf)
Download compressed PostScript version (digitalAudio171.ps.gz)
Download PDF `4 up' version (digitalAudio171_4up.pdf)
Download compressed PostScript `4 up' version (digitalAudio171_4up.ps.gz)
Copyright © 2019-10-15 by Tamara Smyth.
Please email errata, comments, and suggestions to Tamara Smyth<trsmyth@ucsd.edu>
![]() , what is the frequency of the sinusoid?
, what is the frequency of the sinusoid?
![\includegraphics[scale=.8]{eps/QSampSine1}](img17.png)
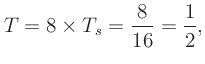
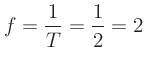 Hz
Hz![]()
![]() , then the frequency is higher:
, then the frequency is higher:
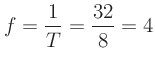 Hz
Hz![]()