Determining the Modulation index

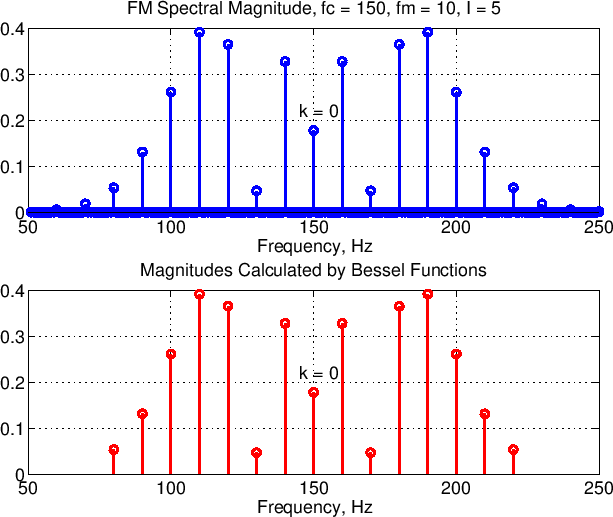
It is possible to determine the exact amplitude of the sidebands using the help of bessel functions.

We can also make use of a rule of thumb for how to set the modulation index $I$ to obtain a frequency deviation $d$ about the the carrier frequency:

$$I = \frac{d}{f_m},$$

where $f_m$ is the frequency of the modulating oscillator.

When $d=0$, the index $I$ is also zero, and no modulation occurs. Increasing $d$ causes the sidebands to acquire more power at the expense of the power in the carrier frequency.

The deviation $d$ acts as a control on FM bandwidth.

The actual amplitude of each sideband can be determined by bessel functions.