The equation of motion for the damped system is obtained by
adding the drag force into the equation of motion:
or alternatively
where
 and
and
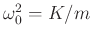 .
.
The damping in a system is often measured by the quantity  , which
is the time for the amplitude to decrease to
, which
is the time for the amplitude to decrease to 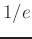 :
:
When a simple oscillator is driven by an external force 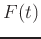 ,
the equation of motion becomes
,
the equation of motion becomes
``MUS 206: Modeling Acoustic Tubes and Wind Instrument Bores/Bells''
by Tamara Smyth,
Department of Music, University of California, San Diego (UCSD).
Download PDF version (wind.pdf)
Download compressed PostScript version (wind.ps.gz)
Download PDF `4 up' version (wind_4up.pdf)
Download compressed PostScript `4 up' version (wind_4up.ps.gz)
Copyright © 2019-05-22 by Tamara Smyth.
Please email errata, comments, and suggestions to Tamara Smyth<trsmyth@ucsd.edu>
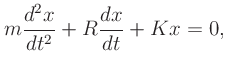
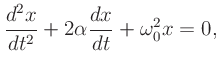
![]() , which
is the time for the amplitude to decrease to
, which
is the time for the amplitude to decrease to ![]() :
:
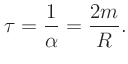
![]() ,
the equation of motion becomes
,
the equation of motion becomes
