Modulation Index

The function 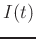$I(t)$, called the modulation index envelope, determines significantly the harmonic content of the sound.

Given the general FM equation

$$x(t) = A(t)\left[\cos(2\pi f_ct + I(t)\cos(2\pi f_mt + \phi_m) + \phi_c\right],$$

the instantaneous frequency 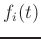$f_i(t)$ (in Hz) is given by

$$f_i(t) = \frac{1}{2\pi}\frac{d}{dt}\theta(t)$$

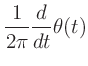
$$= \frac{1}{2\pi}\frac{d}{dt}\left[2\pi f_ct + I(t)\cos(2\pi f_mt + \phi_m) + \phi_c\right]$$

$$= \frac{1}{2\pi}[2\pi f_c - I(t)\sin(2\pi f_mt + \phi_m)2\pi f_m +$$

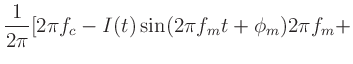
$$\qquad \qquad \frac{d}{dt}I(t)\cos(2\pi f_m t + \phi_m)]$$

$$= f_c - I(t)f_m\sin(2\pi f_mt + \phi_m) +$$

$$\qquad \qquad \frac{1}{2\pi}\frac{d}{dt}I(t)\cos(2\pi f_mt + \phi_m).$$