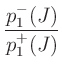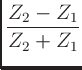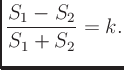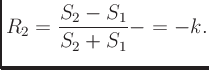Deriving the Reflection Coefficient

**Figure 6:** Scattering: a junction with another section.
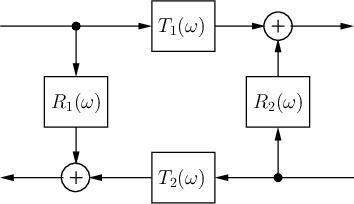$\begin{figure}\centerline{% \input{scattering.pstex_t}}\end{figure}$

The relationship between the adjacent impedances causes a reflection in the first section that can be expressed in terms of tube area cross-sectional ,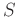

$\displaystyle R_1$	$\displaystyle =$	$\displaystyle \frac{p_1^{-}(J)}{p_1^{+}(J)}$
	$\displaystyle =$	$\displaystyle \frac{Z_2 - Z_1}{Z_2 + Z_1}$
	$\displaystyle =$	$\displaystyle \frac{S_1 - S_2}{S_1 + S_2} = k.$

It follows that the reflection in the second section is

$\displaystyle R_2 = \frac{S_2 - S_1}{S_2 + S_1}- = -k.$

``MUS 206: Modeling Acoustic Tubes and Wind Instrument Bores/Bells'' by Tamara Smyth, Department of Music, University of California, San Diego (UCSD).

Download PDF version (wind.pdf)
Download compressed PostScript version (wind.ps.gz)
Download PDF `4 up' version (wind_4up.pdf)
Download compressed PostScript `4 up' version (wind_4up.ps.gz)